Emilien Azéma
Université de Montpellier, France
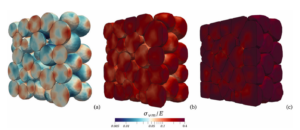
When hard grains (i.e., with high stiffness relatively to the applied stress) are compressed inside a box, they tend to rearrange themselves in a more compact way until a permanent contact forces network is reached, meaning the end of the compression. This “jammed state” depends on the morphology of the grain and the inter-grain friction. What happens now if we consider grains that can deform when the applied stress becomes significant? The compression continues beyond the jammed state, mainly due to the change in shape of the grains and, to a lesser extent, due to their rearrangements. As a result, the solid fraction exceeds that of the Random Close Packing, and it would even be possible to entirely fill the space. By means of coupled finite-discrete elements methods (the non-smooth contact dynamics method NSCD) and experiments using high-resolution imaging coupled with a dedicated DIC algorithm, we analyze the compressive behavior, beyond the jammed state, of two- and three-dimensional granular assemblies made of soR (hyperelastic) grains of different shapes and soft/rigid grain mixtures. We characterize the evolution of the main mechanical observables, from the global scale down to the strain field inside each deformable grain. At the microscopic scale, different power-law relations are evidenced between the local grain structure and contacts, and the packing fraction. We develop an equation that describes the evolution of the packing fraction as a function of the applied pressure. This laUer is derived from the granular stress tensor, its limit to small deformations, and the evolution of the connectivity. This compaction equation provides good predictions from the jamming point up to very high densities without the need for tuning any parameters both for numerical and experimental data.